Читать книгу Heron’s Triangles and Resonance Decays in Lobachevsky Velocity Space - - Страница 3

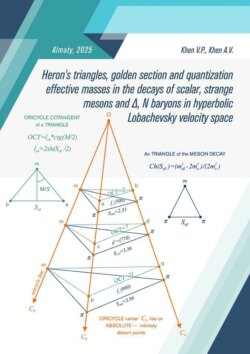
The ends of the velocity vectors of the decay resonance particles are represented by material points of the velocity space located inside a sphere of radius C (C is the speed of light, the points are assigned the rest masses of the decay particles) [2, 9].

The Lorentz group defines in the velocity space the Lobachevsky geometry of curvature k = -1/ C² [1—2]. Material points inside a sphere of radius C represent the Beltrami model of hyperbolic Lobachevsky velocity space (HLVS) [2,7].

Fig_1. Separate ellipses of decay oricycles of ƒ₀ (500), ρ(775), ƒ₀ (980) mesons, combined into one oricycle with the center

at the point “C₀“ of the Absolute.

In the Beltrami model the straight lines and planes represent the straight lines and planes of HLVS [7]. The sphere of radius C, called the Absolute, represents infinitely distant points of HLVS.

A pair of material points of 2-part resonance decay in HLVS can be connected by a straight line segment and an arc of zero curvature line, called the oricycle [7,10]. All oricycles are congruent, just as straight lines in Euclidean space are congruent. In Fig_1, in the plane “π”, “π”, “B” (“B” is the velocity of the beam particle B), individual ellipses of oricycles of scalar mesons, whose centers “C₀” lie on the Absolute, combined into one oricycle with a common center “C₀”.

Archimedes’ laws of levers define a 3rd velocity point “m” on the oricycle arc, in which the role of the lever forces is played by the rest masses of the decay particles, and the lever arms are equal to the oricycle arcs. Thus, to the two material points “π”, “π” with rest masses m_π, a 3-rd velocity point “m” with an additive mass (m